Drawing Five Heads

Setup:

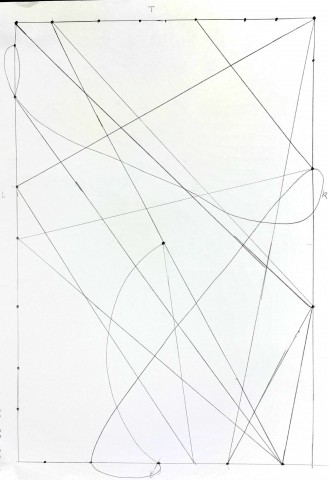
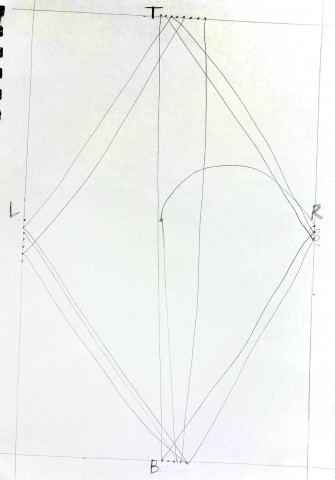
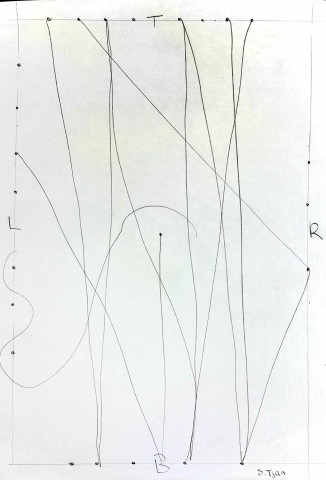
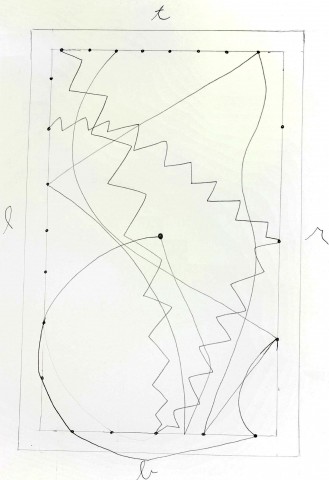
-Hold your paper landscape style.

-Draw two vertical lines down the entire paper measuring 4.25 inches in and 6.75 inches in.

-Draw horizontal lines going across the entire paper measuring 3 inches down and 5.5 inches down.

-Draw within the cross you’ve created.

-On a computer search for images of head proportions, have this in front of you.

 

Before you start:

-There is going to be a head in the center of the cross.

-There will be two heads on the side of that head facing outwards, both in the center of the space on each side.

-There will be a head viewed from beneath at the top of the cross and a head viewed from above at the bottom of the cross. The heads should be tilted enough that they fit in the space.

-Each head should take up as much space as possible without drawing outside of the lines.

-The heads include their necks.

-You can start with any head.

-Each head is the same person.

-Each head should be consistent with the rest of the heads.

 

Drawing the Heads:

-You cannot choose family when choosing models, you can choose celebrities, and you can look up pictures for reference.

-Imagine someone that you have looked up to at some point in your life.  Give the heads their jawline.

-Imagine someone who looked up to you in your life. Give the heads their hair.

-Imagine someone who you can always laugh with. Give the heads their ears along with any piercings.

-Imagine someone that has hurt you in your life, with or without meaning to. Give the heads their lips.

-Imagine someone who has helped you in your life, or someone who coached you. Give the heads their nose and all the marks or freckles in the middle of the face.

-Imagine someone you are or once were in love with. Give the heads their eyes, eyebrows and any wrinkles or marks around their eyes and forehead.

-Imagine someone you used to be really close to. Give the heads their cheeks and cheekbones, along with any skin blemishes or makeup.

-Imagine someone who is lost in life. Give the heads their neck and any jewelry they wear.

-Erase lines drawn in setup

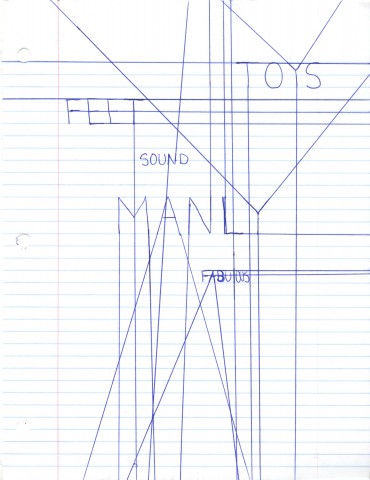
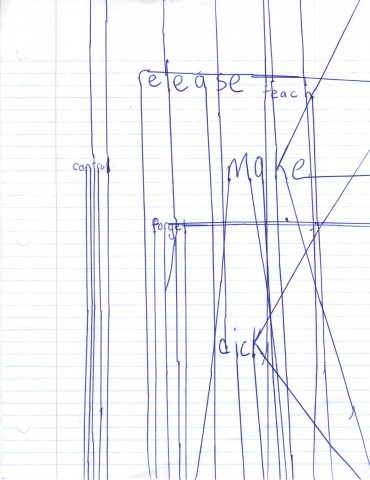
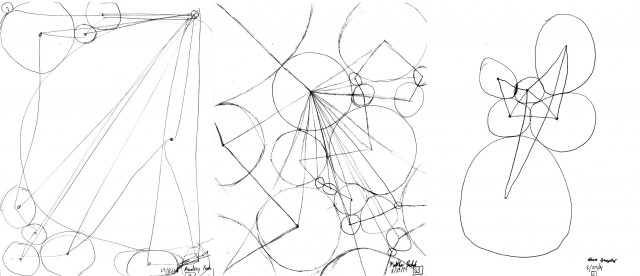
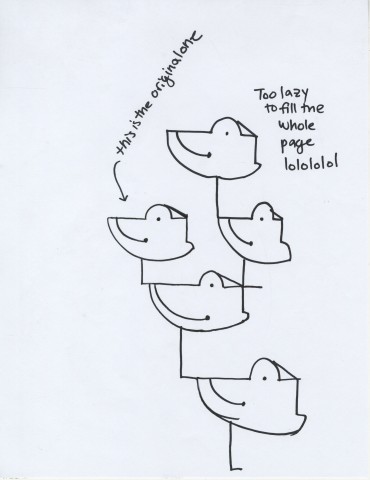
Thank you (in order) David Gordon, anonymous, and Will Taylor for the drawings.

I liked that people enjoyed drawing my instructional drawing, I’ve got feedback that was: “It made me think about people I haven’t thought about in a long time” The interactions between the drawing are strong. These incredibly personal portraits of people are looking at each other whether you stack the drawings vertically or horizontally. I should have never asked my subjects to draw the necks, it emphasized the ending point of the boundary lines I gave, which I wanted to be invisible in the drawing.

According to the designing manifesto, the process is the product. I was really interested in the process of creating drawings, one that no matter who followed the instructions the result would be different and when working with my own drawing instructions that was my focus. I wanted to make instructions that no matter who followed them each piece would be different. Below are the set of instructions I created for my instructional drawings. I also wanted to make the instructions as easy to follow as possible so when writing them, I attempted to separate different aspects required in the piece, from the initial set up to the actual drawing.

Concept:

This project looks to create a series of drawing based off instructions

You will be provided with a pencil, a ruler and a clear sheet of paper

Instructions

On the clear sheet of paper Create a Frame

1. STEP 1

Label the top of the page T

Label the bottom of the page B

Label the left side of the page L

Label the right side of the page R

Draw 2 horizontal lines

Draw the first line ½ an inch from the Bottomof the page

Draw the second line ½ an inch from the Topof the page

Draw 2 vertical lines

Draw the first line ½ an inch from the Leftside of the page

Draw the second line ½ an inch from the Right sideof the page

 

Create a Drawing

STEP 2

With your pencil make dots

-Make a dot in the center of your page, equidistant from the horizontal and vertical lines you drew

Make 8 dots on the horizontal line [next to the label T]

Make 5 dots on the horizontal line [next to the label B]

Make 7 dots on the vertical line [next to the label L]

Make 3 dots on the vertical line [next to the label R]

STEP 3

Draw:

Draw a line (without your ruler) from:

*DO NOT LIFT YOUR PENCIL BETWEEN MOVING FROM DOT TO DOT

2^nd dot next to label T to 3^rd dot next to label R

 =2T to 6R

  4^th dot next to label B to 8^th dot next to label T

=4B to 8T

  3L to halfway between 3B and 4B

Draw a line from your current point to the dot in the center

 Draw a semi-circle from the center and loop through any three dots in on the lines

–5B to 3R

–connect the first dot on the horizontal line on the Top zig zag to an odd dot directly opposite on the Bottom and from that connect it to an even dot directly opposite it on the vertical line. Repeat this process until the painting is finished.

-If you’ve reached this point the painting is finished.

 

One instruction that I initially left ambiguous is the last instruction that says “Repeat this process until the painting is finished”. This instruction is my form of a loop but one that takes into consideration the artist. For normal loops one, or at least a computer, would continue repeating the process until a specific set of parameters are met. As an artist, painting kind of seems like a loop, except for me I never seem to know when to stop. A drawing or painting in my mind is never really finished and so when creating this ‘loop’ I wanted the artist to decided when they thought the drawing was finished, or if they would continue forever. Below is a series of drawing. The first is a drawing a created myself based off of my own instructions. The next three are the drawings I gave out for other people to complete. What I found interesting about the resulting pieces is how each individual interpreted different instructions. I made two initially random instructions. First, I didn’t  dictate where on the lines the people following the instructions would have to place their dots which meant that no matter what the lines made by different artists would never meet or be the same. Second, in one instruction I allowed the artist to connect the dots they selected to any other they selected. This was another way to intentionally generate randomness. One instruction that I found particularly interesting was the zigzagging from one dot to the next. In one painting the person choice to make zigzags, mini ones all the way to the next dot. It’s always things like this that you can’t really anticipate but also serve to create really unique and interesting pieces. That piece was my favorite because it was so unexpected.

My Drawing following the instructions I made

 

First Person to follow my instructions Second Person to follow my instructions Third Person to follow my instructions. My favorite.

Instructions:
1: Draw one of the following (Square, circle, triangle, heart)
2: draw another one, overlapping the first . The same shape cannot overlap itself.
3: repeat step 2 until you run out of room or get bored .

I didn’t exactly have an end result in mind when I gave this to people to do, I just wanted to see what happened. When left to their own devices, there is no consistency in what any of my participants did. One of them decided to stop when they got bored; the other two went until they ran out of space. Two of them generated shapes randomly, the third made theirs into some kind of fractal, probably because they’re a math major and that’s how they roll. Pretty much all of them asked if shapes had to overlap/if a shape could be completely inside another shape or if it had to cross lines with another, so probably I would include some clarification in the instructions of that. I also varied the subjects (one writing major, one engineer, and one math) so I could see how different types of people interpreted the instructions.

My instructional drawing was playing primarily with time spent not drawing. My subjects were given this set of instructions

1. Acquire a Timer
2. Start Timer
3. Draw A Line
4. Draw A Line
5. Draw A Line
6. Draw A Line
7. Draw A Line
8. Stop Timer
9. Write Time Shown on Timer on Paper

While they performed these instructions, I timed the sum total of how long their pencil was in contact with the paper. I used their time and mine to calculate the total time spent not drawing, which I inserted into their drawings below their own times.

 

I was quite surprised by how much variance there was in timing, and how differently people approached the issue of being told to simply draw a line 5 times.  Some consider the lines as individuals, and these take longer than those who simply take them as a set to be swiftly finished.

My initial attempt at the instructional drawing was a flop, partly because the outcome drawing wouldn’t necessarily be able to represent what the instructional was instructing was doing and partly because the commands just aren’t easy for a human to execute without being frustrated (myself included) and partly, just being obsessive. Luckily, the ‘failure’ served as a funny sort of re-whipping-into-shape.

During the first attempt, I found myself getting more into the ruler than the pencil. So in my second attempt, I tried to express that idea much more simply, along with implementing a couple simple loops/conditionals that punish the person executing the instructions for giving negative feedback about their user experience [as a computer].

So while my second attempt was under-parameterized, but perhaps more accessible/’interesting,’ my first attempt ended up failing in a very different way, in that it was too time consuming for the human “computers,” highly parameterized, and pretty devoid of interest. But aside from that, writing pseudocode for human computers was just really fun to do.

So I thought I’d share both attempts, if only to show what not to do (at least if you don’t have a reason to).

 

Second Attempt at Instructional Drawing (the one I actually got people to execute):

m0:
All instructions contained within functions labeled m(z) pertain to all subsequent functions labeled d(x) and n(y).

m2:
For all functions labeled d(x), use only your dominant hand.

m3:
For all functions labeled n(y), use only your non-dominant hand.

m4:
Simply execute each of the following functions in the numerical order listed below, satisfy its conditions, and then then move on to the next function, unless it is otherwise specified by a preceding function or unless x=y, then perform functions d(x) and n(y) simultaneously.

n0:
Hold your pencil in your dominant hand and ruler in your non-dominant hand. Place the paper directly in front of you on the table in portrait orientation.

d1:
Sit in a rolling chair facing a desk with the chair’s height adjusted to be just low enough to fit your knees underneath the desk but no lower.

n2:
Place the ruler on the paper such that its centimeter edge touches both the upper right hand corner and lower left hand corner of the paper.

d3:
Touch the index finger of your hand to the centimeter edge of the ruler at its 2 inch mark. Raise your finger a centimeter above the ruler’s surface.

d4:
Imagine a straight line orthogonal to the cm edge of the ruler (so, parallel to the unit notches) crossing over the 2 inch mark on the ruler.

n5:
Actually trace this imaginary line with your ‘finger’ — move it forward in space right over the ruler’s surface, and then rest it on the ruler’s surface at the midpoint of the imaginary line from function d4.

d5:
Hey! Your ‘finger’ should still line up with the 2 inch mark on the ‘ruler.’ If counting variable [n5b-count] is even, proceed to function n5b, else skip forward to function n6.

n5b:
Turn the paper and ruler 90′ clockwise. Repeat function n5, but replace the word ‘finger’ with ‘pencil’ or vice versa if it was already ‘pencil’. Add 1 to counting variable [n5b-count].

n6:
Apply just enough, but not too much pressure downwards at the 2 inch mark on the ruler to allow you to swivel the other end of the ruler around with your hand.

d7:
Don’t move at all, but imagine swiveling the ruler with one hand while holding it down with the other without disturbing the paper underneath at all.

n8:
Swivel the ruler lightly in a clockwise direction. Proceed to the next function, but don’t stop swiveling.

d9:
Hold the pencil upright with its tip at the midpoint of the long centimeter edge of the ruler. Let the pencil be pushed and guided by the swiveling ruler, generating lines and arcs on the paper.

n9:
Begin to bounce in your chair, while still using your pencil and ruler on the paper to make marks. Increase bounce frequency by a factor of 2 every 10 seconds. If [line-segs] > 1, stop bouncing after (20 + [line-segs]) seconds, else stop bouncing after 20 seconds and skip to m5.

m5:
Count the number of intersections made by the lines you have drawn on the page. Update the value of [line-segs] to this number.

m6:
Please rate the quality of this user experience with a value from 1-9 with 9 being the highest posible rating.
Store this number in the variable [value]
set the variable [satisfation] = [value]
set the value of [satisfaction = x
set the counter variable [overyet?] = [overyet? + 1]
if [overyet?] > 5 AND [satisfaction] >= 10
stop and quit
else
start again at the function d(x).
return pencil, return ruler

 

First Attempt at Instructional Drawing (plus some of the other statements I wrote trying to do this at first..)

#The subject codes like “subject1”, next to the subtask names, were just one idea that then got scrapped#

subtask0: subject1,
before you begin to do this task, sit in a rolling chair facing a desk with the chair’s height adjusted to be just low enough to fit your knees underneath the desk but no lower. Subject2, place one 8.5″ by 11″ piece of white printer paper directly in front of Subject 1 on the desk with the bottom edge of paper flush with the edge of the desk facing Subject1 and also with the midpoint of the line formed by the paper’s bottom edge aligned exactly at the midpoint of Subject1’s torso. Place a ruler and pencil on the table three inches to the right of the paper.

subtask1a: subject1,
pick up the pencil with your dominant hand or either hand if you’re ambidextrous. On the bottom edge of the piece of paper, use the provided ruler to measure out the midpoint of the line formed by the bottom-left corner and bottom right corner of the paper, and very faintly mark this midpoint with an approx. 2 mm. long vertical tick mark. Next, align the ruler so as to make a straight line bisecting the sheet of paper in half vertically through the midpoint you just measured. Using the ruler as a guide, very faintly draw a straight vertical line rising from the bottom of the page to the top. Put the ruler back where it was originally — to the right side of your desk.

subtask1b: subject1,
Grasp your pencil again. Prepare to sign your signature according to the following instructions. Make sure to center your signature at the midpoint of the bottom edge of the paper, such that the faint vertical line you drew also bisects your signature neatly. Use the bottom edge of the paper as a line for you to form your script/letters upon, like you were writing on college-ruled lined paper. After signing, remove your pencil from the paper. Get the ruler again. Measure the height of your signature and divide by two in order to find the midpoint between its highest and lowest points. Make small, faint, horizontal tick marks at both points as guides for the measurement. Then line up the the notched edge of the ruler directly on this horizontal line that bisects your signature, othogonal to the first line. Use your ruler to make the following marks with your pencil: a first endpoint dot 2mm to the left of the signature and another 2mm to the right of the signature, both falling on this bisection line. Using the ruler’s edge to connect these two endpoints, draw a straight line from one to the other, bisecting your signature horizontally.

subtask2a, subject1,
##if executed carefully correctly, subtask2 and subtask3 and subtask4 will analyze and visualize a subset of points that forms part (all the intersections) of the curving line of your signature by evaluating the spatial properties of these points using logical criteria.##

In order to pick an initial point on the curve for evaluation, first compare the height of the highest points on the curve on either half of your bisected signature. In whichever half has the highest point overall, find the lowest and rightmost point of intersection on that half of the curve that is still above the horizontal axis bisecting the signature from subtask1. If the lowest and rightmost point of intersection has not already been filled in, this will be the point you will evaluate as you now proceed to subtask2b.

subtask2a-mini-1, subject1,
[lohi-count] is a counting variable for the number of times that any newly visualized point that you have filled in / marked falls inbetween any two others, but only along the x-axis. To determine the first component of the output of subtask2b — the relational x-axis coordinates defined by the variable [lohi-choice] — which can be either valued as [highest] or [lowest], return [lohi-choice] = [lowest] if [lohi-count] is even, and return [lohi-choice] = [highest] if [lohi-count] is odd.

subtask2a-mini-2, subject1,
[lr-count] is a counting variable for the number of times that any newly visualized point that you have filled in / marked falls inbetween any two others, but only on the y-axis. To determine the second component of the output of subtask2b — the relational y-axis coordinates defined by the variable [lr-choice] — which can be either valued as [leftmost] or [rightmost], return [lr-choice] = [rightmost] if [lr-count] is even, and return [lr-choice] = [leftmost] if [lr-count] is odd.

subtask2b, subject1,
If the lowest and rightmost point has already been filled in, to determine the next point to evaluate, call subtask2a-mini-1 which outputs into [lohi-choice], and then call subtask2a-mini-2 which outputs into [lr-choice], whose respective values replace the contents of the array [lohi-choice, lr-choice]. Proceed to subtask2c. Else, proceed to subtask4. ##The array [lohi-choice, lr-choice] encodes four possible relational spatial codes: [highest, rightmost], [highest, leftmost], [lowest, leftmost], and [lowest, rightmost].

##Subject1 should interpret the values in [lohi-choice, lr-choice] as a command to find the lowest OR highest AND leftmost OR rightmost point of intersection on their signature curve, which will be further evaluated in subtask3 and subtask4 and potentially filled in during subtask4.##
Proceed to subtask2c.

subtask2c, subject1
If you have already filled in the point defined by the current values of [lohi-choice, lr-choice] generated by subtask2b, then choose the nearest point of intersection to your assigned one, but in the opposite direction of the vector defined by [lohi-choice, lr-choice], else call subtask4 [#notsure#]. If the point defined by the previous (one iteration before the current) values of [lohi-choice, lr-choice] generated by subtask2b is a point of intersection between the curve of the signature and either one or both of the two perpendicular lines that bisect it, then temporarily switch (until your current point coordinates fall on these axes again, then switch back) to choosing the furthest point of intersection from your assigned one rather than the nearest one, and in the same direction as the vector described by [lohi-choice, lr-choice]: this output will be interpreted as the new point that you will re-evaluate in subtask3. Proceed to subtask3.

subtask3, subject1,
Visually find the point of interest indexed by the relational coordinate values from subtask2. In evaluating this point using the following set of logical conditions, make sure to FIRST identify the correct truth values — [True] or [False] — and SECOND decide on the corresponding action based on the combination of *ALL* the relevant logical conditions: (1) that you have already filled in this point on the curve; (2) that this point is either the highest or lowest point on the curve whose location is the furthest to either the left or right. If both conditions (1) and (2) are satisfied, stop and procede to the next subtask. If the point on the curve being evaluated does not meet condition (1), but meets condition (2), then run subtask4. Or if the point being evaluated meets condition (1), but not (2), then run subtask2b. Else, run subtask2b.
##A single iteration of this subtask outputs one point’s spatial position on the curve and generates a physical index of it. However, you will test a number of points to see in what order they optimally fulfil a simple set of logical conditions. As you iterate this subtask more, it visualizes in higher resolution the differences in *how well* specific points along the curve meet the set of logical conditions above, and discretizes the ‘raw’ data of the hand-drawn curve.##

subtask4: Mark this point on the curve as accurately as possible with a single dot with the tip of your pencil. If you have evaluated every point on the line above the azimuth/horizontal axis of the signature’s curve or if there is not enough room physically on the line to draw any more points, then stop and procede to the next subtask. If not, run subtask2b.

 

subtask2: subject1, you will visualize a subset of points that forms part of the curving line of your signature by evaluating the spatial properties of these points using logical criteria. *ALL* of the logical conditions that make up the following set must be met fully in order to complete the subtask properly: (1) that you have not already marked this point on the curve with a dot; (2) that this point is the highest point on the curve whose location is the furthest to the left. If condition (1) and (2) are both met, mark this point as accurately as possible with a single dot with the tip of your pencil. Stop and procede to the next subtask. If the point on the curve being evaluated does not meet condition (1), but meets condition (2); or if the point being evaluated meets condition (1), but not (2), then repeat subtask2 until a point is found that meets both conditions (1) and (2). If you have evaluated every point on the line above the azimuth/horizontal axis of the signature’s curve or if there is not enough room physically on the line to draw any more points, then stop and procede to the next subtask.A single iteration of this subtask outputs one point’s spatial position on the curve and generates a physical index of it. However, you will test a number of points to see in what order they optimally fulfil a simple set of logical conditions. As you iterate this subtask more, it better visualizes the differences in *how well* specific points along the curve meet the set of logical conditions above, and discretizes the ‘raw’ data of the hand-drawn curve.

subtask3: mark this point as accurately as possible with a single dot with the tip of your pencil.

subject1, pick up ruler with your other hand. With your other hand, position the notched edge of the ruler so that this dot directly touches the 2 inch mark on the ruler’s notched edge. Return your pencil to where it originally was on the desk — to the right of the paper.

subtask5:

Touch the index finger of your non-dominant hand to the notched edge of the ruler at its 2 inch mark. Raise your finger a centimeter above the ruler’s surface. Imagine a straight line transversing the surface of the ruler (parallel to the unit notches), which connects one of its long edges to the other. Actually trace this imaginary line with your finger, moving it forward in space right above the ruler’s surface, and then stopping at the line’s midpoint. Your finger should still line up with the 2 inch mark. Apply just enough, but not too much pressure downwards to allow you to swivel the opposite end of the ruler with your other hand. Try swiveling the ruler with one hand while holding it down with the other without disturbing the paper underneath at all. Now find the the highest rightmost point on the curve created in subtask1 and swivel the ruler so that the notched edge just touches this point, forming an imaginary line segment between the highest leftmost and rightmost points on the curve. Lift both hands of the ruler without disturbing the paper or ruler’s positions. Grasp the pencil in your dominant hand again. Press down on the ruler with your non-dominant hand without shifting its position and without letting your fingers overlap the notched edge of the ruler. Mark a single dot at the highest leftmost point on the curve. Place your pencil tip exactly on the first dot generated in this subtask, and apply pressure sideways against the edge of the ruler in order to steady the pencil tip. Draw a straight ruler-guided line from this first, left dot to the other dot made in subtask2. Stop. Remove pencil from the paper.

Subtask6: subject1, visually identify the longest line segment remaining on the paper that was generated exclusively during subtask2. If you visually identify that there is only one line segment on the paper that was the longest generated during subtask2, then mark a single dot with the tip of your pencil at the midpoint of this line segment. ##Use your brain to encode the information that your making a mark at the midpoint of a line segment is called ‘line bisection’ and that ‘line bisections’ generate two new ‘line segments’ of equal lengths.## Stop and proceed to the next subtask. Mark a single dot with the tip of your pencil at the midpoint of this line segment in order to bisect it into two new line segments of equal lengths. If you visually identify multiple line segments of equal length on the paper that were the longest generated during subtask2, then mark a single dot with the tip of your pencil at the midpoint of only the leftmost one of these line segment, bisecting it. Stop and proceed to the next subtask.

Subtask7: subject1, #use your brain to encode the information that there is a variable called ‘num-lb’ that you will use to represent the cumulative number of ‘line bisections’ that you have executed exclusively during subtask2, subtask3, and subtask4. Use your brain to encode the information that there is another variable called ‘num-ls’ that you will use to represent the cumulative number of ‘line segments’ on the paper that you have generated exclusively during subtask2, subtask3, and subtask4. To calculate the current value of ‘num-lb’, you must first calculate the current value of ‘num-ls’, and then subtract 1 from this value.# Calculate the current value of ‘num-lb’. If ‘num-lb’ is equal to or more than , repeat subtask3 ‘num-lb’ times, else stop and proceed to the next subtask. ‘num-lb’

subtask3: subject1, place pencil to paper marking a single dot at the midpoint of the line created in subtask2, Repeat subtask3 the midpoint of the left segment and

then repeat subtask1 in the space directly above the first signature by vertically stack each finished iteration of the signature directly on top of the previous, taking care to make sure that the lowest regions of the line of the current iteration just touch the highest of the previous, neither hovering above not intersecting to create overlap. Continue to stack signatures until the very top edge of the paper is reached and then stop. If you must vertically compress the final iteration of the signature to fully fit within the page, do so. After the final iteration, stop. Repeat the whole

Subject 2: Simultaneously, starting from when Subject 1 lifts their pen from page after completing the fifth iteration, pull Subject 1’s rolling chair backwards orthogonally to the the desk in front — at a rate of 1 step backwards (foot1-behind-foot2-repeat) per blink of Subject 2’s eyes.

[lohi-choice] = [highest], then
[lr-choice] = [rightmost]
(with each call of subtask2b, count the # of times [lohi-count] that any new visualized point falls inbetween any two others only on the horizontal axis and [lohi] is even, choose lowest and if it is odd, choose highest) AND leftmost OR rightmost (with each call of subtask2b, count the # of times [lr-count] that any new visualized point falls inbetween any two others only on the horizontal axis and [lr-count] is even, choose rightmost and if it is odd, choose leftmost) point of intersection and this will be the point you evaluate. Proceed to subtask3.

INSTRUCTIONAL DRAWING

 

My instructions were simple enough, and elicited varied responses from my three volunteers. I think I should have left the line extension step more vague, so as to vary interpretation more. Regardless, I got what I expected aesthetics wise. The first two volunteers left a bit more empty space than I expected, which I actually like very much.

Chris Copeland, my roommate Kasem Kydd, was eating pie in my room Charlotte Faye, person in this class

Here are the actual instructions provided to other students:

1. Draw a small circle anywhere on the page, and draw a dot at the center of this circle.
2. On any point on the circumference of this circle, draw a larger circle such that the circumference of this new circle is tangent to the circumference of the previous circle. Make sure that the new circle does not overlap with any other circles. Draw a dot at the center of this new circle.
3. Repeat step 2 until not possible. Keep count of the number of circles drawn – the goal is to draw as many as circles as possible.
4. Draw a line from the center of the last circle to the center of the previous circle.
5. Repeat step 4 until the first circle is reached. (At the end, every line should be connected to at most 2 other lines.)
6. Draw a line from the center of every circle drawn to the center of the very first circle drawn.
7. On the bottom right of the page, sign your name, date, and also write down the total number of circles drawn. Draw a box around the total number of circles drawn.

Here are images of the results:

(From left to right: Audrey Yeoh’s interpretation, Matt Sebek’s interpretation, and Brent Strysko’s interpretation.  Thank you all for being such excellent test subjects – er, art assistants.)

In retrospect, I think my instructions must have been rather confusing. Although I kindly informed my participants that I could not answer any queries about exactly what my instructions are asking them to do, every participant expressed a certain level of frustration regarding exactly what the end what supposed to look like. Although each acknowledged a certain lack of artistic ability (particularly when relating to drawing circles that are supposed to be more circular than blob-like), I was somewhat surprised at the difference in what I imagined the result to be and exactly what resulted. I understand that while my participants are not computers, it was somewhat interesting to see them execute the task considering each of them were from engineering backgrounds. Despite this, I was pleased by the level of randomness that occurred from unintended ambiguities from my instructions. If I were to do this again, I would attempt to gather people of different backgrounds and I would also add one more step at the beginning of my instructions: 0) Read all instructions before attempting to continue to step 1.

Firstly, a big thanks to everyone who volunteered to create an instructional drawing. All participants are credited by name in the caption of their respective drawing(s).

All participants were given an 8.5 x 11 sheet of paper, a fine point black sharpie, and asked to choose between two sets of instructions, hereafter known as “bird” and “squiggy”.

Interestingly, three out of the five people who volunteered first tried the “bird” instruction set, and then asked to try the “squiggy” instruction set. (The other two stuck with their initial choice.)

Why the switch? Well, here’s the instruction set for “bird”:

Begin by drawing the top half of a circle with the diameter of half an inch.

Place a dot in the center of where the half-circle’s full circle would be.

On an edge of the half-circle that is parallel with the dot, draw an acute angle so that each of the two lines creating the angle is the length of the half-circle’s radius, and so that the interior of the acute angle faces the dot.

From the edge of the acute angle that is not connected to the half-circle, draw a half-inch long straight vertical line that travels away from the dot.

Continued from the straight line, draw a quarter-circle that mirrors the half-circle.

Go back to the half-circle. At the side opposite from the acute angle, draw a horizontal line that is one inch long, and travels away from the dot.

From the end of the one inch horizontal line to the end of the quarter-circle, draw and arc.

Find the intersection between the half-circle and the one inch line. Move vertically away from this point by a half-inch, and place a new dot.

Draw an arc between the new dot and the three fourths of an inch point of the one inch line.

Starting from the quarter-inch point on the one inch line, move down until you encounter the edge of the shape. At this edge, draw a half-inch vertical line traveling away from the shape.

At a right angle to the half inch line (180 degrees on the unit circle), draw a horizontal quarter-inch line.

This is now a finished shape. You must continue making this shape until the page is full, with the condition that you may only draw this shape where the right angle quarter-inch line can connect to a previously drawn shape.

Compared to the instruction set for “squiggy”:

Draw a squiggly shape that is a closed space and does not have overlapping lines.

Fill this shape with horizontal lines. The lines may not pass through the outline of the shape.

Fill this shape with vertical lines. The lines may not pass through the outline of the shape.
Repeat until page is full of squiggly shapes filled with horizontal and vertical lines.

Repeat until page is full of squiggly shapes filled with horizontal and vertical lines.

As “bird” is a bit hard to visualize, below is a digitally-assisted image to help make sense of the instructions.

Miranda Jacoby’s (digitally assisted) Bird Drawing

Now for the submissions.

Sean Reidy’s Bird Drawing Meghan Chin’s Bird Drawing Natalie Moss’ Bird Drawing Sylvia Kosowski’s Bird Drawing

Sylvia sums up the general sentiment toward the “bird” instructions quite nicely. Natalie took a rather clever approach and cut her drawing out of its page, creating a new page that the drawing filled. Overall, it seems like everyone had trouble understanding how the beak shape and/or the wing shape was supposed to work. The idea of “bird” was to define a shape relative to points and shapes already plotted. Evidently I have to work on my ability to describe the overall orientation of those shapes in an understandable way.

Now for the “squiggy” drawings:

Kay Nestor’s Squiggy Drawing Sean Reidy’s Squiggy Drawing Meghan Chin’s Squiggy Drawing Natalie Moss’ Squiggy Drawing

Again, no two submissions are alike. Kay seemed focused on making her shapes into recognizable objects, while Sean realized that the kind of line was not specified, and thus included dotted lines and a number line into his drawing. Unlike “bird”, “squiggy” is a lot more open-ended, allowing for more organic permutations.

Overall, the instructional drawing exercise was a sliding scale between frustration and fun.